Study of the $ {{a_1}}$(1260) resonance in the $ {{\gamma p \to \pi^+\pi^+\pi^- n}} $ reaction

The $ a_1(1260) $ resonance with quantum numbers $ J^{PC} = 1^{++} $ is a candidate for the chiral partner of $ \rho $ meson [1-3]. It is also described as a $ q \overline{q} $ state in the Numbu-Jona-Lasino model [4, 5]. Apart from the quark model, it is considered as a gauge boson of the local hidden symmetry [6, 7]. By using the chiral unitary approach, $ a_1(1260) $ is a state arising from the interactions of pairs of hadrons in coupled channels [8, 9]. In addition, the nature of $ a_1(1260) $ has also been investigated using the $ \tau $ decay spectrum into three pions [10-12], and multi-pion decays of light vector mesons [13, 14]. Recently, the $ a_1(1260) $ resonance was studied in Ref. [15] in the decay of $ \tau \to \nu_{\tau} \pi^- a_1(1260) $ through a triangle mechanism.

The dynamically generated nature of $ a_1(1260) $ has been tested in the radiative decay process. The decay of $ a_1(1260) $ into $ \pi \gamma $ in Ref. [16] was also studied in Refs. [17, 18] and found to be in agreement with the experimental data if $ a_1(1260) $ is associated with the dynamically generated picture. In Ref. [19], the lattice result for the coupling constant of $ a_1(1260) $ into the $ \rho\pi $ channel is similar to the one obtained in Ref. [8]. Recently, the production of $ a_1(1260) $ in the $ \pi^-p \to a_1(1260)^-p $ reaction within the effective Lagrangian approach was studied in Ref. [20] based on the results of [8]. Besides, it was found that the elementary $ q\bar{q} $ component of $ a_1(1260) $ is comparable to the hadronic composite [21-23]. Using the chiral unitary approach, the large $ N_{\rm c} $ behavior of the $ a_1(1260) $ state was investigated in Ref. [22], and it was found that $ q\bar{q} $ is not the main component of $ a_1(1260) $.

Based on the values obtained by two different experimental groups [24, 25], it is estimated that the mass and Breit-Wigner width of $ a_1(1260) $ is $ M_{a_1(1260)} $ = 1230 $ \pm $ 40 MeV and $ \Gamma_{a_1(1260)} = (250-600) $ MeV, respectively [26]^①. The large uncertainties of the mass and width of $ a_1(1260) $ in the Particle Data Group (PDG) [26] show that the knowledge of $ a_1(1260) $ is very limited. Therefore, a study of $ a_1(1260) $ photoproduction could be helpful to determine the mass and width of this resonance.

Meson photoproduction off a proton provides one of the most direct platforms to extract information about the hadronic structure [27, 28]. We should point out that in the experiments, no signal representing $ a_1(1260)^+ n $ photoproduction [29-33] could be isolated even though the $ \pi \gamma $ radiative width of $ a_1(1260) $ very likely exceeds that of $ a_2(1320) $ [16, 34-36]. The absence of the $ J^{PC} = 1^{++} $ state in charge exchange photoproduction is puzzling. In this paper, by investigating the $ \gamma p \to a_1(1260)^+ n $ process within the $ \pi $-exchange mechanism, we calculate its total cross-section. The $ \pi^+\pi^+\pi^- $ mass distribution and the total cross-section of $ \gamma p \to \pi^+\pi^+\pi^- n $ are studied. In addition, we consider the non-resonance contributions to the $ \gamma p \to \pi^+\pi^+\pi^- n $ resonance, which involve nucleon pole terms. Other contributions, which involve $ \Delta(1232) $ and nucleon excited states, can be removed based on the $ \pi^+n $ invariant mass spectrum from the experiments [33].

The article is organized as follows. After the introduction, we present the reaction mechanism of $ a_1(1260) $ photoproduction. The possible background relevant to the production of $ a_1(1260) $ is discussed and the $ \pi^+\pi^+\pi^- $ mass distribution is presented in Sec. 3. This work ends with a discussion and conclusion.

In this section, we discuss the $ a_1(1260) $ production mechanism. Fig. 1 shows the basic tree-level Feynman diagram for the $ a_1(1260) $ production in the $ \gamma p \to $$ a_1(1260)^+ n $ reaction via the $ \pi $-exchange process.

Figure 1.  Feynman diagram for the $\gamma p \to {a_1}{\left( {1260} \right)^ + }n$ reaction via $ \pi $-exchange.

For the $ \pi NN $ vertex, we adopt the commonly used effective Lagrangian

where the standard value, $ g_{\pi NN}^2/{4\pi} = 14.4 $, is adopted as in Refs. [37, 38]. In addition, the form factor is introduced for suppressing the vertex coupling when one or two interacting particles go off-shell. For the $ \pi NN $ vertex, the form factor satisfies the relation

where $ \Lambda_{\pi} $ is a cut-off parameter [39, 40], which will be discussed in the following. $ q_{\pi} $ is the momentum of the exchanged $ \pi $ meson.

The vertex depicting the interaction of $ a_1(1260) $ and $ \pi \gamma $ is [17, 18]

where $ \varepsilon_{\mu}(p_{a_1}) $ and $ \varepsilon_{\nu}(p_{\gamma}) $ are the polarization vectors corresponding to $ a_1(1260) $ and photon, respectively.

With the vertex above, we can easily get the partial decay width of $ a_1\to \pi \gamma $,

where $ M_{a_1} = 1230 $ MeV is the nominal mass of $ a_1(1260) $. Using the partial decay width $ \Gamma_{a_1\to \pi \gamma} = 640\pm246 $ keV of $ a_1(1260) $ as listed in PDG [26], we get $ g_{a_1\pi\gamma} = 244 \pm 94 $ MeV, where the error is from the uncertainties of $ \Gamma_{a_1\to \pi \gamma} $ and the mass of $ a_1(1260) $. In the following calculations, we take the average value $ g_{a_1\pi\gamma} = 244 $ MeV.

With the above integrants, one can get the scattering amplitude of the $ \gamma(p_1) p(p_2) \to a_1(1260)^+(p_4)+ n(p_3) $ process as

By defining $ s = (p_1+p_2)^2 $, the corresponding unpolarized differential cross-section reads

where $ \theta $ is the scattering angle of $ a_1^+ $ meson relative to the beam direction in the c.m. frame, while $ \vec{p}_1^{\ {\rm c.m.}} $ and $ \vec{p}_4^{\ {\rm c.m.}} $ are the three-momenta of the initial photon and the final $ a_1^+ $, respectively.

In Fig. 2, the solid, dashed and dotted lines are obtained with $ \Lambda_{\pi} = 1.0 $, 1.3 and 1.6 GeV, respectively. From Fig. 2 one can see that the total cross-section via $ \pi $ exchange increases very rapidly close to the threshold, and the peak position of the total cross-section is $ E_{\gamma} \sim 2.6 $ GeV. The total cross-section is proportional to $ g_{a_1\pi\gamma}^2 $, which indicates that the cross-section is proportional to the partial decay width $ \Gamma_{a_1\to \pi \gamma} $. Since the exact value of $ \Gamma_{a_1\to \pi \gamma} $ is not determined by theory or experiment, in this work we take $ \Gamma_{a_1\to \pi \gamma} = 640 $ keV. The result is comparable with the cross-section of $ a_2(1320) $ photoproduction [41].

Figure 2.  Dependence of the total cross-section of $\gamma p \to {a_1}{\left( {1260} \right)^ + }n$ as a function of E_γ.

Next, we consider the $\gamma p\to a_1(1260)^+n\to\rho^0\pi^+n\to $$ \pi^+\pi^+\pi^-n $ and $ \gamma p\to \rho^0p\to\pi^+\pi^+\pi^-n $ processes. Here $ \gamma p\to \rho^0p\to\pi^+\pi^+\pi^-n $ can occur via the nucleon pole term [42].

The $ \gamma p\to a_1(1260)^+n\to\rho^0\pi^+n\to\pi^+\pi^+\pi^-n $ reaction with $ \pi $ exchange is shown in Fig. 3, where the relevant kinematic variables are shown. As discussed in the introduction, we take the coupling of $ a_1(1260) $ to the $ \rho \pi $ channel as obtained in Ref. [8].

Figure 3.  Feynman diagram for the $ \gamma p \to {a_1}{\left( {1260} \right)^ + }n \to {\rho ^0}{\pi ^ + }n \to$$ {\pi ^ + }{\pi ^ + }{\pi ^ - }n$ reaction via $\pi$ exchange.

The $ a_1^+\rho^0\pi^+ $ vertex can be written as

where $ \varepsilon_{a_1} $ and $ \varepsilon $ are the polarization vectors of $ a_1(1260) $ and $ \rho $, respectively. $ g_{a_1\rho\pi} $ is the coupling of $ a_1(1260) $ to $ \rho\pi $. We take $ g_{a_1\rho\pi} = (-3795+{\rm i}2330) $ MeV as obtained in Ref. [8], where only the $ S $-wave interaction was considered. Note that there is also a $ D $-wave contribution to the $ a_1\rho\pi $ vertex as investigated in Ref. [43], where the $ D $-wave contribution was found to be small.

For the vertex of $ a_1(1260)^+ $ interacting with $ \rho^0\pi^+ $, we also introduce a form factor $ F_{a_1\rho\pi} $, which is

with a typical value of $ \Lambda_{a_1} = 1.5 $ GeV as in Refs. [20, 44].

The $ a_1(1260) $ propagator is

where the width $ \Gamma_{a_1} $ is dependent on its four-momentum squared, and we can take the form as in Refs. [45, 46],

where $ \Gamma_{3\pi} $ is the decay width for the process $a_1(1260)\to $$ \rho\pi \to 3\pi $ [44], and $ \Gamma_0 $ is the decay width for the other processes. Following the experimental result in Ref. [24] for the total decay width of $ a_1(1260) $, we take $ \Gamma_{0} = 201 $ MeV for $ \Gamma_{a_1} = 367 $ MeV at $ \sqrt{q_{a_1}^2} = 1230 $ MeV.

For the structure of the $ \rho \pi \pi $ vertex, we use the general interaction as,

where $ <> $ stands for the trace in $ SU(3) $ , and $ g = \displaystyle\frac{m_{V}}{2f} $, with $ m_{V} = m_{\rho} $ , and $ f = 93 $ MeV is the pion decay constant. The $ \rho \pi \pi $ vertex can then be written as

For the vertex of $ \rho $ interacting with $ \pi\pi $, we also introduce a form factor $ F_{\rho\pi\pi} $, which satisfies the relation

with a typical value of $ \Lambda_{\rho} = 1.5 $ GeV as used in Ref. [44].

The $ \rho $ propagator is

where $ \Gamma_\rho $ is energy dependent. Because the dominant decay channel of $ \rho $ is $ \pi \pi $, we take

with $ \Gamma_{\rm on} = 149.1 $ MeV, and

where $ M^2_{\rm inv} = q_{\rho}^2 = (p_6+p_7)^2 $ or $ (p_5+p_7)^2 $ is the invariant mass squared of the $ \pi^+ \pi^- $ system. We take $ m_\rho = 775.26 $ MeV in this work.

It is worth to mention that the parametrization of the width of $ \rho $ meson shown in Eq. (15) is meant to take into account the phase space of each decay mode as a function of the energy [40, 47, 48]. In the present work we take explicitly the phase space for the P-wave decay of the $ \rho $ into two pions.

We finally obtain the scattering amplitude for the diagram shown in Fig. 3,

Besides the resonance contribution of the $ a_1(1260) $ resonance, we study another kind of reaction mechanism for the $ \gamma p \to\pi^+\pi^+\pi^-n $ reaction, which is depicted in Fig. 4, where we have considered the contribution from $ \gamma p \to\rho^0 p \to\pi^+\pi^-\pi^+n $. In Fig. 4, the relevant kinematic variables are also shown.

Figure 4.  Feynman diagram for the $\gamma p \to {\rho ^0}{\pi ^ + }n \to {\pi ^ + }{\pi ^ + }{\pi ^ - }n$ reaction via $\pi $ exchange.

To compute the contribution of Fig. 4, we take the interaction density for $ \rho \gamma \pi $ as [49, 50],

where $ A_{\beta},\pi $ and $ \rho_{\nu} $ denote the fields of the photon, $ \pi $ and $ \rho $, respectively. The coupling constant $ g_{\rho\gamma\pi} $ can be obtained from the experimental decay width $ \Gamma_{\rho^0 \to \pi^0\gamma} $ [26] , which leads to $ g_{\rho\gamma\pi} = 0.76 $.

Other vertexes are the same as given above. With the above preparation, we get the transition amplitude for the diagram shown in Fig. 4,

with

where $ \Lambda_{\pi} = 0.6 $ GeV and $ \Lambda_{N} = 0.5 $ GeV are taken from Refs. [49, 50, 51]. This choice of the cut-off leads to a satisfactory explanation of the $ \rho^0 $ photoproduction at low energies. Note that the value of $ \Lambda_{\pi} $ is different from the one we used for the $ \gamma p \to na_1(1260)^+ $ production. Other cut-off parameters are the same as given above.

The total cross-section of the $ \gamma p\to\pi^+\pi^+\pi^-n $ reaction can be obtained by integrating the invariant amplitude in the four-body phase space:

with

where 2! is a statistical factor for the final two $ \pi^+ $ mesons, and the four-body phase space is defined as [26]

where $ \vert\vec{p}_6^{\ *a}\vert $ and $ \Omega_6^{*a} $ are the three-momentum and solid angle of the out-going $ \pi^+ $ in the c.m. frame of the final $ \pi^+\pi^- $ system, $ \vert \vec{p}_5^{\ *b} \vert $ and $ \Omega_5^{*b} $ are the three-momentum and solid angle of the out-going $ \pi^+ $ in the c.m. frame of the final $ \pi^+\pi^+\pi^- $ system, and $ \vert \vec{p}_3 \vert $ and $ \Omega_3 $ are the three-momentum and solid angle of the out-going $ n $ in the c.m. frame of the initial $ \gamma p $ system. In the above equation, $ M_{\pi^+\pi^-} $ is the invariant mass of the $ \pi^+\pi^- $ two body system, and $ M_{\pi^+\pi^+\pi^-} $ is the invariant mass of the $ \pi^+\pi^+\pi^- $ three body system, and $ s = (p_1+p_2)^2 $ is the invariant mass squared of the initial $ \gamma p $ system.

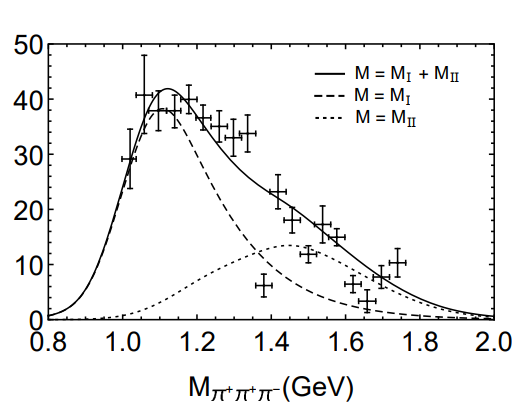
In Ref. [33], the $ \gamma p\to\pi^+\pi^+\pi^-n $ reaction was studied in the photon energy range $ 4.8-5.4 $ GeV. The 3$ \pi $ mass distributions are measured from the $ 1^{++}(\rho\pi)_S $ partial wave. In Fig. 5, we show the theoretical results, $ c_1{\rm d}\sigma/{\rm d}M_{\pi^+\pi^+\pi^-} $, for the $ \pi^+\pi^+\pi^- $ invariant mass distributions for the $ \gamma p\to\pi^+\pi^+\pi^-n $ reaction at $ E_{\gamma} = 5.1 $ GeV, compared with the experimental measurements of Ref. [33]. The theoretical results are obtained with $ c_1 = 21.5 $ and $ c_1 = 18 $ for $ M_{a_1} = 1080 $ and $ 1230 $ MeV, respectively, which have been adjusted to the experimental data reported by the CLAS collaboration [33]. From Fig. 5, it is seen that the bump structure around $ 1.4-1.6 $ GeV may account for the nuclear pole contribution. If we use $ M_{a_1} = 1080 $ MeV, the $ \pi^+\pi^+\pi^- $ invariant mass distributions agree well with the experimental data. On the other hand, the theoretical results with $ M_{a_1} = 1230 $ MeV can not describe the bump structure around 1.1 GeV.

Figure 5.  The 3 $\pi$ invariant mass spectrum for the $\gamma p\to \pi^+\pi^+\pi^-n$ process compared with the data obtained by the CLAS collaboration from the $1^{++}(\rho\pi)_S$ partial wave [33]. Left and right plots correspond to $M_{a_1} = 1080$ and 1230 MeV , respectively.

In addition to the differential cross-section, we also calculated the total cross-section for the $ \gamma p\to \pi^+\pi^+\pi^-n $ process as a function of the photon beam energy $ E_{\gamma} $. The results are shown in Fig. 6, where one can see that the total cross-section increases rapidly near the threshold, and the peak of the total cross-section is at $ E_{\gamma} = 2.5 $ and 2.9 GeV corresponding to $ M_{a_1} = 1080 $ and $ 1230 $ MeV, respectively. The differential and total cross-sections could be checked in future experiments, such as those at CLAS.

Figure 6.  Total cross-section for the $\gamma p\to \pi^+\pi^+\pi^-n$ process. Left and right plots correspond to $M_{a_1} = 1080$ and 1230 MeV , respectively.

In recent years, it has been found that the $ a_1(1260) $ resonance, although long accepted as an ordinary $ q\overline{q} $ state, can be dynamically generated from the pseudoscalar-meson-vector-meson interaction, and therefore qualify as a pseudoscalar-vector molecule. In this work, we have proposed to study the $ a_1(1260) $ resonance in the photoproduction process. Since $ a_1(1260) $ was observed in the radiative decay of $ a_1(1260)^+\to \pi^+\gamma $, the $ \gamma p \to a_1(1260)^+ n $ reaction by $ \pi $ meson exchange is the main process for producing $ a_1(1260) $. Our numerical results show that the total cross-section of $ \gamma p \to a_1(1260)^+ n $ is of the order of 10 μb, which is comparable with the cross-section for photoproduction of $ a_2(1320) $.

In addition, taking the coupling constant obtained from the picture where the $ a_1(1260) $ resonance is a dynamically generated state from pseudoscalar-meson-vector-meson interaction, the $ \pi^+\pi^+\pi^- $ invariant mass distributions from the $ \gamma p \to \pi^+\pi^+\pi^- n $ reaction were studied. With $ M_{a_1} = 1080 $ MeV, we can describe the experimental data for the $ \pi^+\pi^+\pi^- $ invariant mass distributions fairly well. The total cross-section of the $ \gamma p \to \pi^+\pi^+\pi^- n $ reaction was also studied using the model parameters determined from a comparison with the experimental data for the $ \pi^+\pi^+\pi^- $ invariant mass distributions. It is expected that our model calculations could be tested by future experiments with the $ \gamma p\to \pi^+\pi^+\pi^-n $ reaction at the photon beam energy $ E_{\gamma} $ around 2.5~2.9 GeV.

One of us (Xu Zhang) would like to thank Yin Huang for helpful discussions.